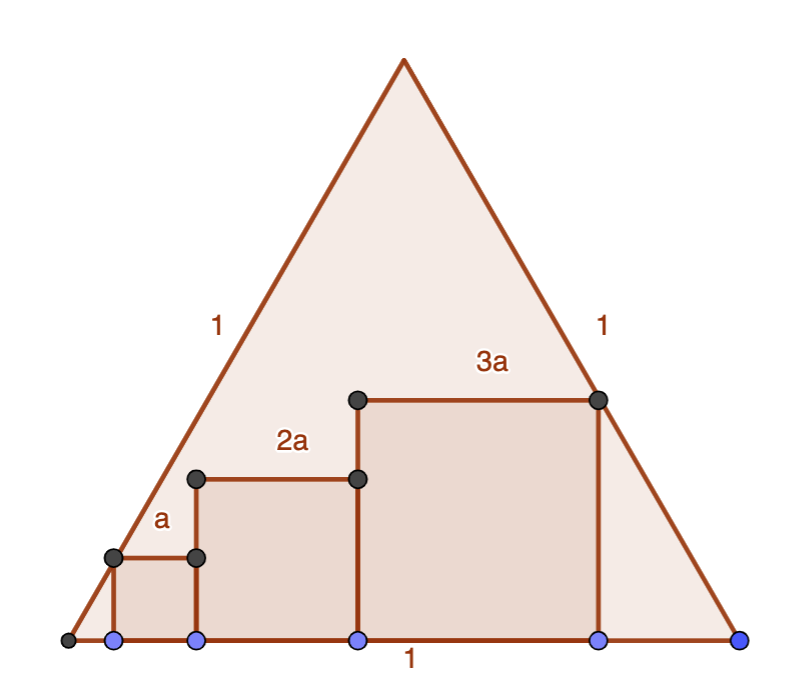
Puzzle time - A little basic geometry
-
||I had wanted to solve it without using trigonometry, and found that I had to resort to using the Pythagorean theorem to find the ratio of a/x, which is what tan(60°)=sqrt(3) is anyway. I ended up with a=1/(6+4/sqrt(3))≈0.12||
@Axtremus said in Puzzle time - A little basic geometry:
I had to resort to using the Pythagorean theorem
Picking a nit here, but you assume that the lines intersect at right angles. That's not stated in the diagram, is it? It's an assumption on your part.
-
@Axtremus said in Puzzle time - A little basic geometry:
I had to resort to using the Pythagorean theorem
Picking a nit here, but you assume that the lines intersect at right angles. That's not stated in the diagram, is it? It's an assumption on your part.
@George-K said in Puzzle time - A little basic geometry:
@Axtremus said in Puzzle time - A little basic geometry:
I had to resort to using the Pythagorean theorem
Picking a nit here, but you assume that the lines intersect at right angles. That's not stated in the diagram, is it? It's an assumption on your part.
True, but if the lines do not intersect at right angles, then even the trigonometric functions would not be applicable, i.e., using the Pythagorean theorem is not any “more wrong” than using a trigonometric function in this case.
-
@George-K said in Puzzle time - A little basic geometry:
@Axtremus said in Puzzle time - A little basic geometry:
I had to resort to using the Pythagorean theorem
Picking a nit here, but you assume that the lines intersect at right angles. That's not stated in the diagram, is it? It's an assumption on your part.
True, but if the lines do not intersect at right angles, then even the trigonometric functions would not be applicable, i.e., using the Pythagorean theorem is not any “more wrong” than using a trigonometric function in this case.
@Axtremus said in Puzzle time - A little basic geometry:
True, but if the lines do not intersect at right angles, then even the trigonometric functions would not be applicable
That's right. I'm just being an asshole here....
||@jon-nyc would add "again."||